C++实现AVL树

目录

AVL树的概念

二叉搜索树虽可以缩短查找的效率，但如果数据有序或接近有序二叉搜索树将退化为单支树，查找元素相当于在顺序表中搜索元素，效率低下。因此，两位俄罗斯的数学家G.M.Adelson-Velskii和E.M.Landis在1962年发明了一种解决上述问题的方法：当向二叉搜索树中插入新结点后，如果能保证每个结点的左右子树高度之差的绝对值不超过1(需要对树中的结点进行调整)，即可降低树的高度，从而减少平均搜索长度。

一棵AVL树或者是空树，或者是具有以下性质的二叉搜索树：

1. 它的左右子树都是AVL树
2. 左右子树高度之差(简称平衡因子)的绝对值不超过1(-1/0/1)
3. 平衡因子的计算是右子树的高度减去左子树的高度的差值结果

如果一棵二叉搜索树是高度平衡的，它就是AVL树。如果它有n个结点，其高度可保持在O(log N) ，搜索时间复杂度O( log N)。

AVL树节点的定义

template<class K, class V>structAVLTreeNode{
    AVLTreeNode<K, V>* _left;//左孩子
    AVLTreeNode<K, V>* _right;//右孩子
    AVLTreeNode<K, V>* _parent;//父亲结点
     
    pair<K, V> _Kv;//键值int _bf;//平衡因子//构造函数AVLTreeNode(const pair<K, V>& Kv):_left(nullptr),_right(nullptr),_parent(nullptr),_Kv(Kv),_bf(0){}};

AVL树的定义

template<classK,classV>classAVLTree{typedef AVLTreeNode<K, V> Node;public:AVLTree():_root(nullptr){}private:
    Node* _root;};

AVL树的插入

AVL树就是在二叉搜索树的基础上引入了平衡因子，因此AVL树也可以看成是二叉搜索树。那么AVL树的插入

过程可以分为两步：

按照二叉搜索树的方式插入新节点

与根结点比较如果比根大就往右子树插入，如果比根小就往左子树插入，直到走到合适的位置就插入，由于这里是三叉链所以需要处理结点之间的关联关系

boolInsert(const pair<K, V>&kv){if(!_root) _root =newNode(kv);//初始根节点

        Node* cur = _root;
        Node* parent = _root;while(cur){
            K key = cur->_Kv.first;if(key > kv.first)//比根结点的key值小，{
                parent = cur;
                cur = cur->_left;}elseif(key < kv.first)//比根结点的key值大，{
                parent = cur;
                cur = cur->_right;}else{returnfalse;//插入失败}}//开始插入
        cur =newNode(kv);
        Node* newNode = cur;if(parent->_Kv.first > newNode->_Kv.first)//新插入的结点key值比根节点小就插入到左子树{
            parent->_left = newNode;
            newNode->_parent = parent;}else//新插入的结点key值比根节点大就插入到右子树{
            parent->_right = newNode;
            newNode->_parent = parent;}}

调整节点的平衡因子

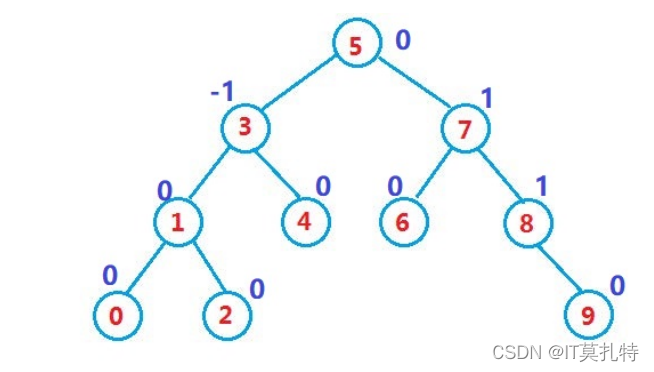
当左右子树的高度发生了变化，那么就需要对父亲及祖先路径上的所有结点的平衡因子进行调整

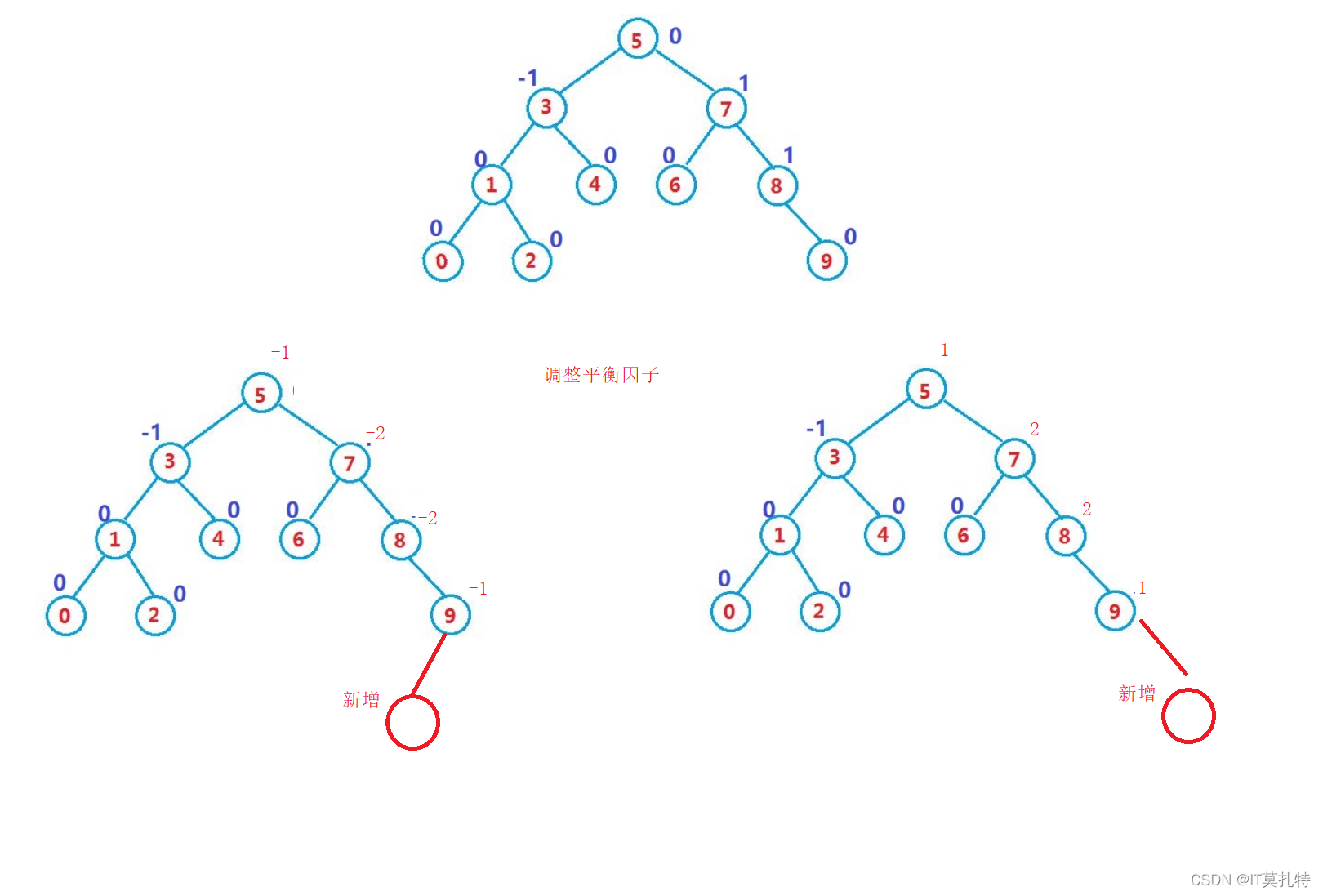
//更新祖先路径的所以结点的平衡因子/* 
            总结五种情况：
                1、新增结点出现在父结点的左边，平衡因子减减
                2、新增结点出现在父结点的右边，平衡因子加加
                3、父亲的平衡因子为0就不再调整
                4、父亲结点的平衡因子为1或者-1继续调整
                5、父亲结点的平衡因子为2或者-2那就旋转
                
        */while(parent){if(parent->_left == cur) parent->_bf--;//1、if(parent->_right == cur) parent++;//2、if(parent->_bf ==0)break;//3、if(parent->_bf ==-1|| parent->_bf ==1)//4、 {
            cur = parent;
            parent = parent->_parent;}if(parent->_bf ==-2|| parent->_bf ==2)//5、{//旋转if(parent->_bf ==-2){if(cur->_bf ==-1)RotateR(parent);//左边高，右单旋elseRotateLR(parent);//左右双旋}else//右 parent->_bf == 2{if(cur->_bf ==1)RotateL(parent);//右边高左单旋转elseRotateRL(parent);//右左双旋}break;}}

AVL树的四种旋转

旋转的原则是遵循搜索树的规则，尽量让两边平衡

如果在一棵原本是平衡的AVL树中插入一个新节点，可能造成不平衡，此时必须调整树的结构，使之平衡化。根据节点插入位置的不同，AVL树的旋转分为四种：

右单旋

新节点插入较高左子树的左侧—左左：右单旋

不管是哪种单旋都得考虑两种情况：
1、局部旋转，如果parent并不是树的_root结点，那么就需要调整subL和根结点的关系
2、独立旋转，parent就是树的_root结点，那么subL就是旋转后的根节点了

3、subLR有可能为null

//右单旋voidRotateR(Node* parent){
    Node* subL = parent->_left;
    Node* subLR = subL->_right;

    parent->_left = subLR;if(subLR) subLR->_parent = parent;//防止subLR为nullptr

    subL->_right = parent;
    Node* parent_parent = parent->_p    arent;//指针备份
    parent->_parent = subL;if(_root == parent)//如果parent就是树的根 {
        _root = subL;//subL取代parent
        _root->_parent =nullptr;}else//如果parent并不是树的根{if(parent_parent->_left == parent) parent->_left = subL;else parent_parent->_right = subL;

        subL->_parent = parent_parent;//subL去做parent_parent的孩子}//调节平衡因子
    subL->_bf = parent->_bf =0;}

左单旋

新节点插入较高右子树的右侧—右右：左单旋

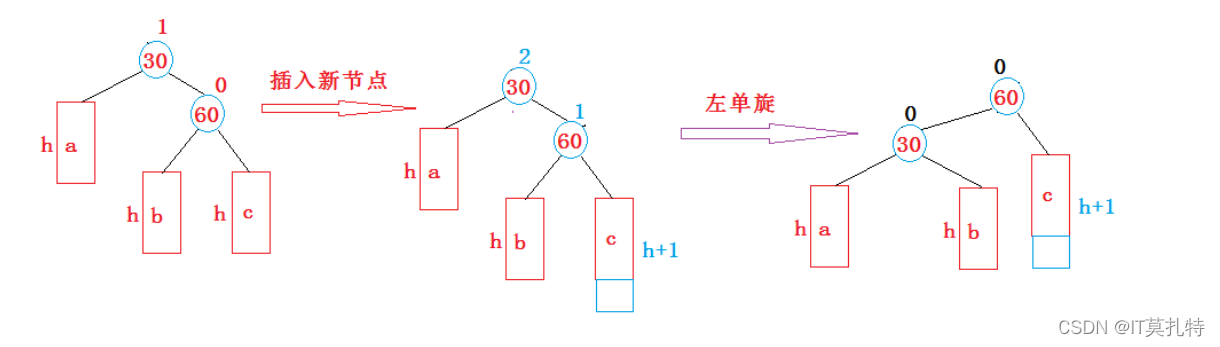
跟右单旋几乎是一样的做法
1、局部旋转，如果parent并不是树的_root结点，那么就需要调整subL和根结点的关系
2、独立旋转，parent就是树的_root结点，那么subL就是旋转后的根节点了
3、subRL有可能为null

//左单旋voidRotateL(Node* parent){
    Node* subR = parent->_right;
    Node* subRL = subR->_left;
    
    parent->_right = subRL;if(subRL) subRL->_parent = parent;
    
    subR->_left = parent;
    Node* parent_parent = parent->_parent;
    parent->_parent = subR;if(_root == parent){
        _root = subR;
        _root->_parent =nullptr;}else{if(parent_parent->_left == parent) parent_parent->_left = subR;else parent_parent->_right = subR;

        subR->_parent = parent_parent;}
    subR->_bf = parent->_bf =0;}

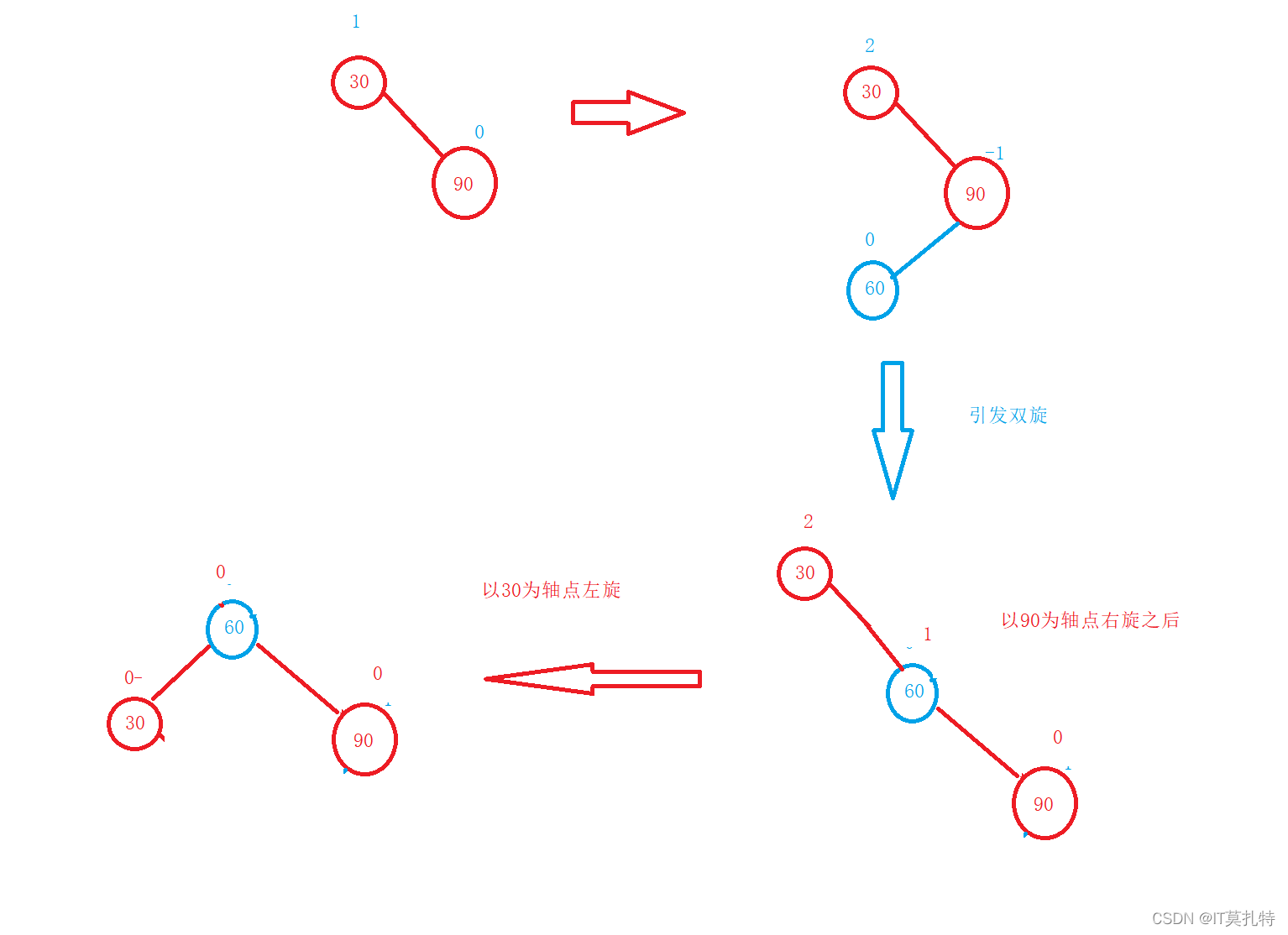
左右双旋

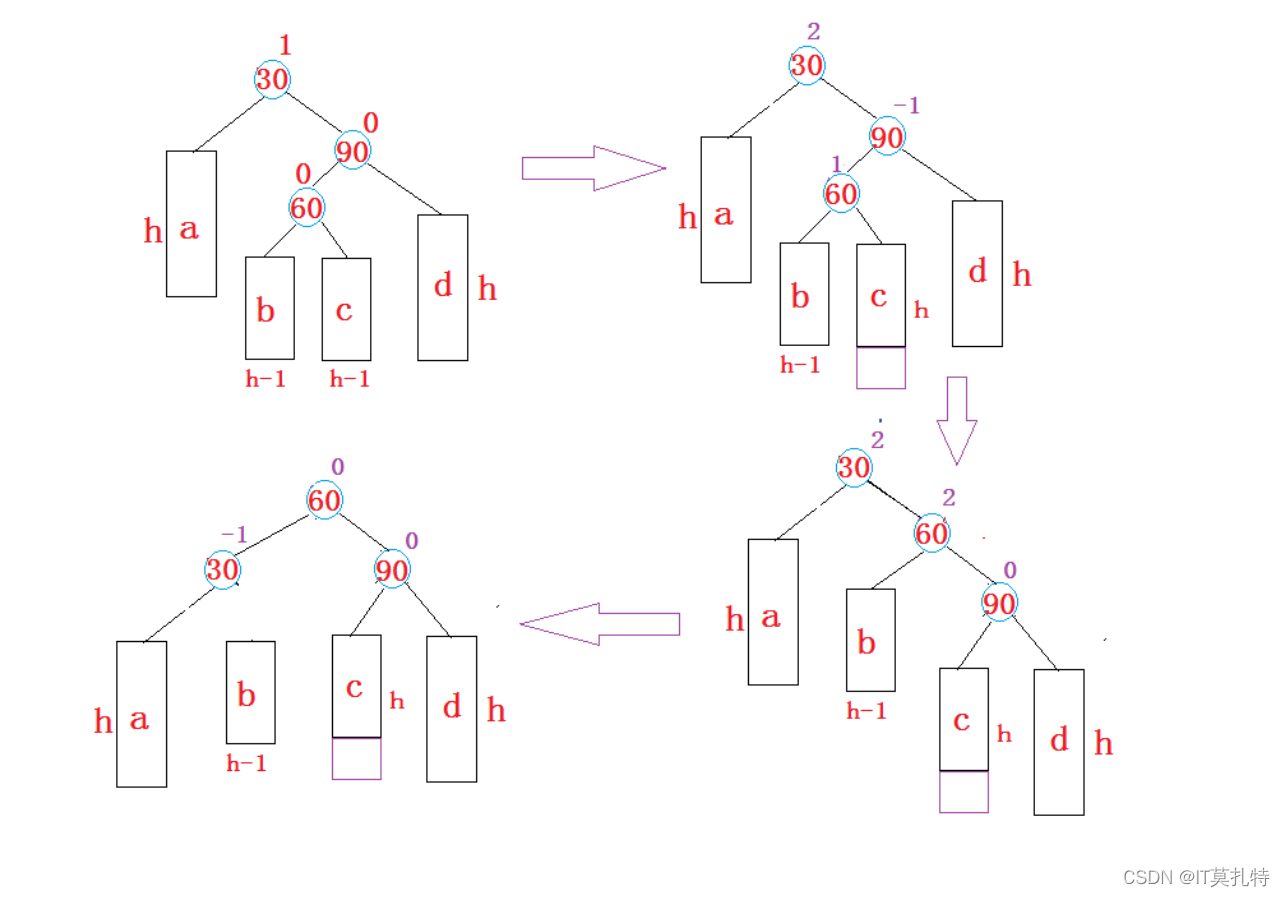
新节点插入较高左子树的右侧—左右：先左单旋再右单旋

1、新增结点在b或c都会影响左右子树的高度，从而引发双旋
h > 0情况一：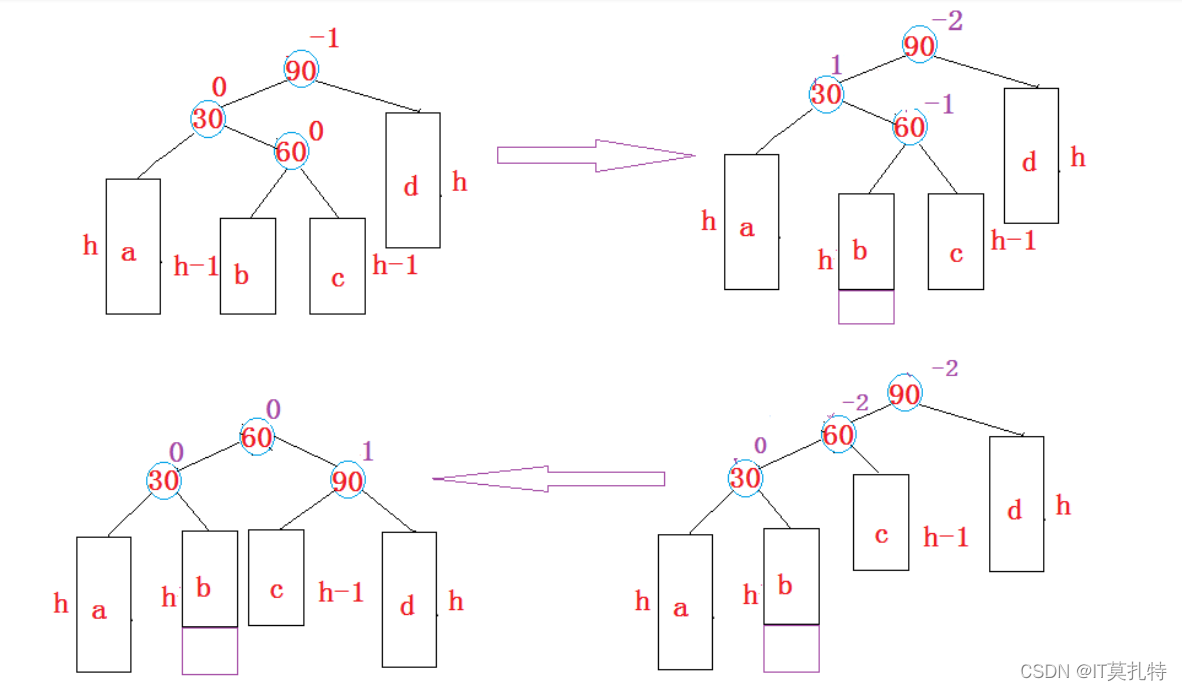

h > 0，情况二：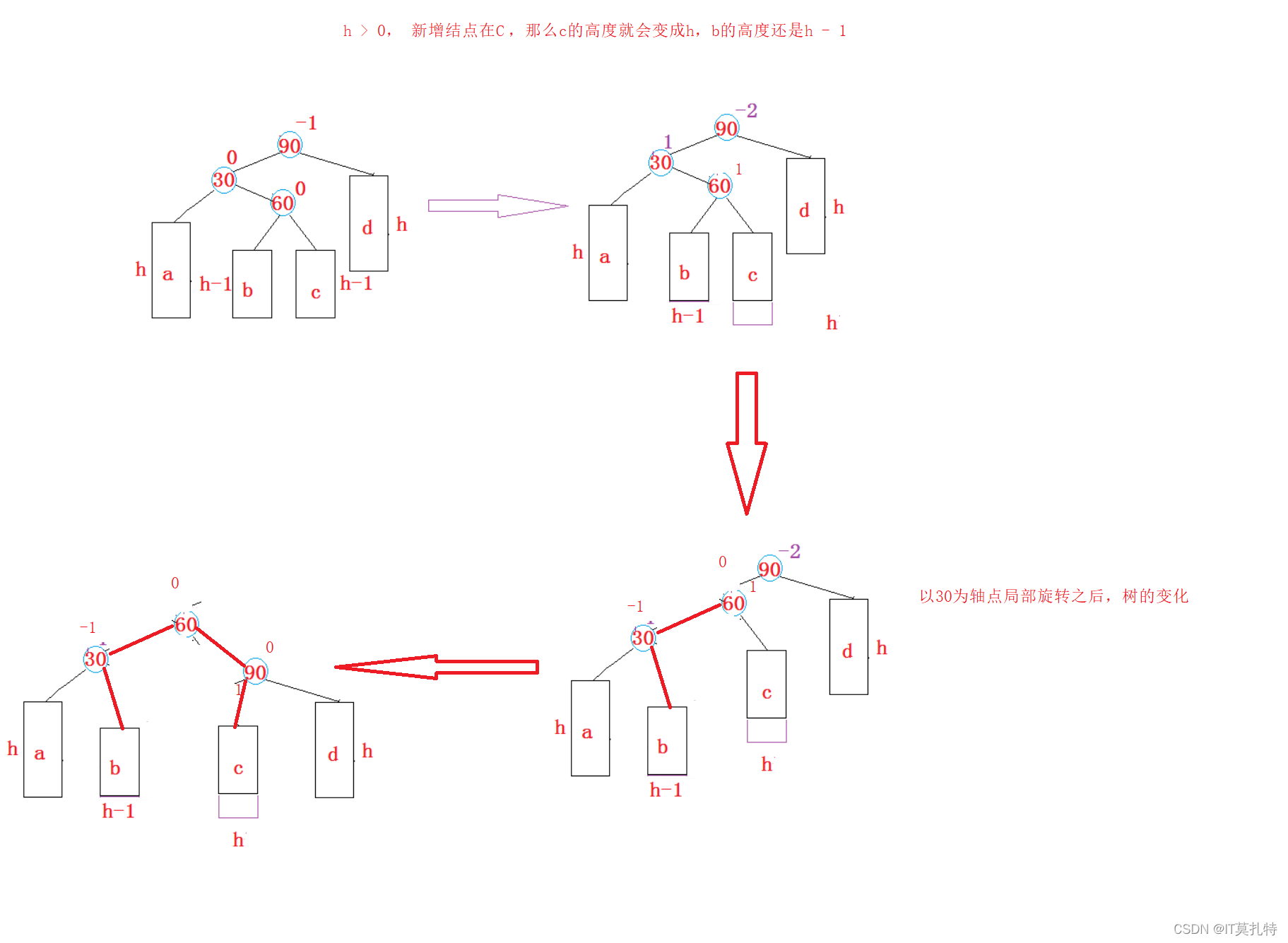

h == 0情况三：

//左右旋转voidRotateLR(Node* parent){
        Node* subL = parent->_left;
        Node* subLR = subL->_right;int bf = subLR->_bf;RotateL(parent->_left);RotateR(parent);if(bf ==-1)//h > 0，新增结点在b{
            parent->_bf =1;
            subLR->_bf =0;
            subL->_bf =0;}elseif(bf ==1)//h > 0，新增结点在c{
            subL->_bf =-1;
            subLR->_bf =0;
            parent->_bf =0;}elseif(bf ==0)//h = 0{
            parent->_bf =0;
            subLR->_bf =0;
            subL->_bf =0;}}

右左双旋

右左双旋跟左右双旋的情况基本是类似的，这里就不列举多种情况了

新节点插入较高右子树的左侧—右左：先右单旋再左单旋

//右左旋转voidRotateRL(Node* parent){
        Node* subR = parent->_right;
        Node* subRL = subR->_left;int bf = subRL->_bf;RotateR(parent->_right);RotateL(parent);if(bf ==-1)//h > 0，新增结点在b{
            parent->_bf =0;
            subR->_bf =1;
            subRL->_bf =0;}elseif(bf ==1)//h > 0，新增结点在c{
            parent->_bf =-1;
            subR->_bf =0;
            subRL->_bf =0;}elseif(bf ==0)//h = 0{
            subR->_bf =0;
            subRL->_bf =0;
            parent->_bf =0;}}

查找

Node*Find(const K& key){
    Node* cur = _root;while(cur){if(key > cur->_Kv.first) cur = cur->_right;//左子树elseif(key < cur->_Kv.first) cur = cur->_left;//右子树elsereturn cur;}}

其他接口

判断是不是平衡二叉树

intheight(Node* root)//求高度{return!root ?0:max(height(root->_left),height(root->_right))+1;}void_Inorder(Node* root)//中序遍历 {if(!root)return;_Inorder(root->_left);printf("%d : %d\n",root->_Kv.first, root->_Kv.second);_Inorder(root->_right);}//判断是不是平衡二叉树boolIsAVLTree(){return_IsAVLTree(_root);}bool_IsAVLTree(Node* root){if(!root)returntrue;int left =height(root->_left);int right =height(root->_right);//检查平衡因子    if(right - left != root->_bf){printf("错误的平衡因子 %d :%d\n", root->_Kv.first, root->_Kv.second);returnfalse;}return(abs(right - left)<2)&&_IsAVLTree(root->_left)&&_IsAVLTree(root->_right);}

析构函数

//析构函数~AVLTree(){Destroy(_root);
    _root =nullptr;}voidDestroy(Node *root)//后序销毁结点{if(!root)return;Destroy(root->_left);Destroy(root->_right);delete root;}

拷贝构造

Node*copy(Node* cp){if(!cp)returnnullptr;

    Node* newnode =newNode(cp->_Kv);
    newnode->_left =copy(cp->_left);
    newnode->_right =copy(cp->_right);return newnode;}//拷贝构造AVLTree(const AVLTree<K, V>& job){if(&job !=this)
    _root =copy(job._root);}

拷贝赋值

voidoperator=(AVLTree<K, V> tmp){if(&tmp !=this)swap(tmp._root,this->_root);}

重载operator[ ]

V&operator[](const K& key){return(Insert(make_pair(key,V())).first)->_Kv.second;}

AVL树的完整实现代码博主已经放在 git.