Java 算法篇-链表的经典算法：有序链表去重、合并多个有序链表

🔥博客主页： 【小扳_-CSDN博客】**
❤感谢大家点赞👍收藏⭐评论✍**

1.0 链表的说明

    **为了更好的讲解本篇当中的两种经典算法，先创建一个带哨兵的链表。链表是一种常见的数据结构，用于存储一系列元素。链表由一系列节点组成，每个节点包含一个数据元素和一个指向下一个节点的指针。**

代码如下：

import java.util.Iterator;

public class List implements Iterable<Integer>{
    private  Node sentry;

    static class Node {
        public int value;
        public Node next;

        public Node(int value, Node next) {
            this.value = value;
            this.next = next;
        }
    }

    public List() {
        sentry = new Node(-1,null);
    }

    //头插节点
    public void addFirst(int value) {
        sentry.next = new Node(value,sentry.next);
    }

    //尾插节点
    public void addLast( int value) {
        Node temp = sentry;
        while (temp.next != null) {
            temp = temp.next;
        }
        temp.next = new Node(value,null);
    }

    //重写迭代器
    @Override
    public Iterator<Integer> iterator() {
        return new Iterator<Integer>() {
            Node p = sentry.next;
            @Override
            public boolean hasNext() {
                return p != null;
            }

            @Override
            public Integer next() {
                int value = p.value;
                p = p.next;
                return value;
            }
        };
    }

}

    **简单讲解一下，创建了一个链表类，该类中包含一个静态内部类，即节点类，还实现了一个基本的方法：头插节点、尾插节点、重写了迭代器等等。**

需要了解的小伙伴可以点击该链接：【Java 数据结构篇-实现单链表核心API-CSDN博客】

2.0 有序链表去重的实现方式

   ** 在此之前，需要分为两个方向：**

** 一、需要保留重复值的节点**

** 使用递归来实现有序链表的去重、使用双指针来实现有序链表的去重。**

** 二、不需要保留重复值的节点**

** 使用递归来实现有序链表的去重、使用三指针来实现有序链表的去重。**

2.1 有序链表去重(保留重复的节点) - 使用递归来实现

    **具体思路：先来考虑终止递出的条件为：p == null 或者 p.next == null ，对于 p == null 情况，当该链表为空链表时，直接返回 null ，对于 p.next == null 情况，当递出到最后只剩一个时，也没有必要继续下去了，不会再有重复的值的节点了。再来考虑递出的具体过程：当 p.value == p.next.value 的情况，就该忽略该节点，则需要返回下一个节点；当 p.value != p.next.value 的情况，就需要返回该节点，但是在返回之前，需要对 p.next 该节点的指向进行重整。**

代码如下：

    //去重方法一(保留):递归
    public List removeRecursion(List list) {
        Node sentry1 = list.sentry;
        sentry = recursion1(sentry1);
        return list;
    }

    private Node recursion1(Node p) {

        if (p == null || p.next == null) {
            return p;
        }
        if (p.value == p.next.value) {
            return recursion1(p.next);
        }else {
            p.next = recursion1(p.next);
            return p;
        }

    }

** 需要注意的是，先得判断链表对象是否为 null ，不然会空指针异常。**

2.2 有序链表去重(保留重复的节点) - 使用双指针来实现

    **具体思路：定义两个指针 n1 与 n2 ，对于 n1 来说：n1 一开始指向头节点，假如指向哨兵节点时，那么后续就会掺入了哨兵节点的值来比较，因此，n1 一开始时需要指向头节点。对于 n2 来说，n2 = n1.next ，也就是 n2 在 n1 指向的节点的前一个节点。接下来：当 n1.value == n2.value 时，则将 n1.next = n2.next ；当 n1.value != n2.value 时，则 n1 = n1.next 。     **

** 循环的条件为：(n2 = n1.next) != null 。**

代码如下：

    //去重方法二(保留):双指针
    public List removeDoublePointer(List list) {
        if (list == null) {
            return null;
        }

        //少于两个节点，不存在重复的值
        if (list.sentry.next == null || list.sentry.next.next == null) {
            return null;
        }

        Node n1 = list.sentry.next;
        Node n2;

        while ((n2 = n1.next) != null) {

            if (n2.value == n1.value) {
                n1.next = n2.next;
            }else {
                n1 = n1.next;
            }
        }

        return list;
    }

    **需要注意的是，先得判断对象是否为 null ；还有一种情况，当节点少于两个时，不存在重复的值的节点。**

2.3 有序链表去重(不保留重复的节点) - 使用递归来实现

    **具体思路：先来考虑递出的终止条件为：当 p == null 或者 p.next == null 的情况时，直接返回 p 该节点，因为当 p.next == null 时，不存在有两个重复值的节点，因此就没有必要再继续递归下去了。再来考虑递出的两种情况：当 p.value != p.next.value 时，没有重复，则返回当前节点 p ，但是在此之前，需要对 p.next 重新赋值，即重新调整 p.next 的指向；当 p.value == p.next.vaule 时，存在重复，则将该值的节点全部找出来，直到找到最后一个节点。循环的条件为： p.value == p.next.value ，循环结束后，得到的 p 就是最后一个重复值的节点，因为不需要这个节点，则返回下一个节点。**

代码如下：

    //去重方法一(不保留):递归
    public List removeRepeat(List list) {
        Node temp = list.sentry;
        sentry = recursion(temp);
        return list;
    }

    public Node recursion(Node p) {
        if (p == null || p.next == null) {
            return p;
        }

        if (p.value != p.next.value) {
            p.next = recursion(p.next);
            return p;
        }else {
            while (p.value == p.next.value) {
                p = p.next;
            }
            return recursion(p.next);
        }

    }

    **同样的，也需要先判断该对象是否为 null ，否则容易报异常。**

2.4 有序链表去重(不保留重复的节点) - 使用三指针来实现

    **具体思路：先定义三个指针，对于 p1 来说： 一开始时指向哨兵节点，假如不实现哨兵节点，则删除不了当链表中前几个为重复值的节点(比如：1->1->1->2->null) ，因此，需要实现哨兵来完成该需求；对与 p2 来说：一开始时指向头节点，即 p1.next；对于 p3 来说：一开始时指向头节点的下一个节点，即 p2.next 。接下来，对于循环的两种过程来分析：当 p2.value == p3.value 时，需要接着找到两个节点的值不相等的时候，所以内层循环条件为：p2.value == p3.value 且 p3 != null，这里需要特别注意的是，千万不能丢了 p3 != null 的限制条件。跳出内层循环是，就可能意味着找到了，则将 p1.next = p3 ；当 p2.value != p3.value 时，直接 p1 = p1.next 即可。外层循环的条件为：（(p2 = p1.next) != null 且 (p3 = p2.next) != null）**

代码如下：

    //去重方法二(不保留):三指针
    public List removeThreePointer(List list) {

        if (list == null) {
            return null;
        }

        Node n1 = list.sentry;
        Node n2 ;
        Node n3 ;
        while ((n2 = n1.next) != null && (n3 = n2.next) != null) {
            if (n2.value == n3.value) {
                while (n3 != null && n2.value == n3.value) {
                    n3 = n3.next;
                }
                n1.next = n3;
            }else {
                n1 = n1.next;
            }
        }
        return list;
    }

    **这里有个小技巧，对与 p2、p3 来说，不着急赋值，在判断条件的时候再进行赋值，可以简略代码量，提高可读性。**

3.0 合并升序链表

   ** 分为两种情况：**

** 一、合并两个升序链表**

** 使用迭代法实现合并链表、使用递归实现合并链表**

** 二、合并多个升序链表**

** 合并多个升序链表就是一个个合并两个升序链表的情况，用递归来实现**

3.1 合并升序链表(两个链表) - 迭代法

    **具体思路：对于两个链表合并来说，在各自的链表中分别定义一个指针，分别指向各自的头节点。合并一条新的链表，定义一个指针指向哨兵节点。**

代码如下：

    //合并升序链表
    public static List combinedList(List l1,List l2) {
        if (l1 == null && l2 == null) {
            return null;
        } else if (l1 == null) {
            return l2;
        } else if (l2 == null) {
            return l1;
        }

        List newList = new List();
        Node node1 = l1.sentry.next;
        Node node2 = l2.sentry.next;
        Node p = newList.sentry;

        while (node1 != null && node2 != null) {

            if (node1.value < node2.value) {
                p.next = node1;
                node1 = node1.next;
            }else {
                p.next = node2;
                node2 = node2.next;
            }
            p = p.next;
        }

        if (node1 != null) {
            p.next = node1;
        }
        if (node2 != null) {
            p.next = node2;
        }
        return newList;
    }

3.2 合并升序链表(两个链表) - 递归法

    **具体思路：先来考虑递出的终止条件为：当 p1 == null 时，则直接返回 p2；当 p2 == null 时，则直接返回 p1。再来考虑递出的过程：当 p1.value < p2.value 时，返回的节点为 p1 节点，在返回节点之前，需要将 p1.next 对该节点的重新调整指向下一个节点；当 p1.value >= p2.value 时，返回的节点为 p2 节点，同理，在返回该节点之前，需要将 p2.next 对该节点的重新调整指向下一个节点。**

代码如下：

     private Node combineRecursion(Node p1, Node p2) {
        if (p1 == null) {
            return p2;
        } else if (p2 == null ) {
            return p1;
        }

        if (p1.value < p2.value) {
            p1.next = combineRecursion(p1.next,p2);
            return p1;
        }else {
            p2.next = combineRecursion(p1,p2.next);
            return p2;
        }

     }

3.3 合并多个升序链表

    **具体思路：这是一个多路递归，在每一次的递归过程中，都可以看成有两个升序链表进行来合并。**

代码如下：

     //实现多个升序链表合并
    public List moreCombine(Node[] nodes) {
        List list = new List();
        list.sentry.next = moreCombineRecursion(nodes,0, nodes.length-1);
        return list;
    }

    private Node moreCombineRecursion(Node[] nodes,int i,int j) {

        if (j == 1) {
            return nodes[i];
        }
        int mid = (i + j) >>> 1;
        Node left = moreCombineRecursion(nodes,i,mid);
        Node right = moreCombineRecursion(nodes,mid+1,j);
        return combineRecursion(left,right);
    }

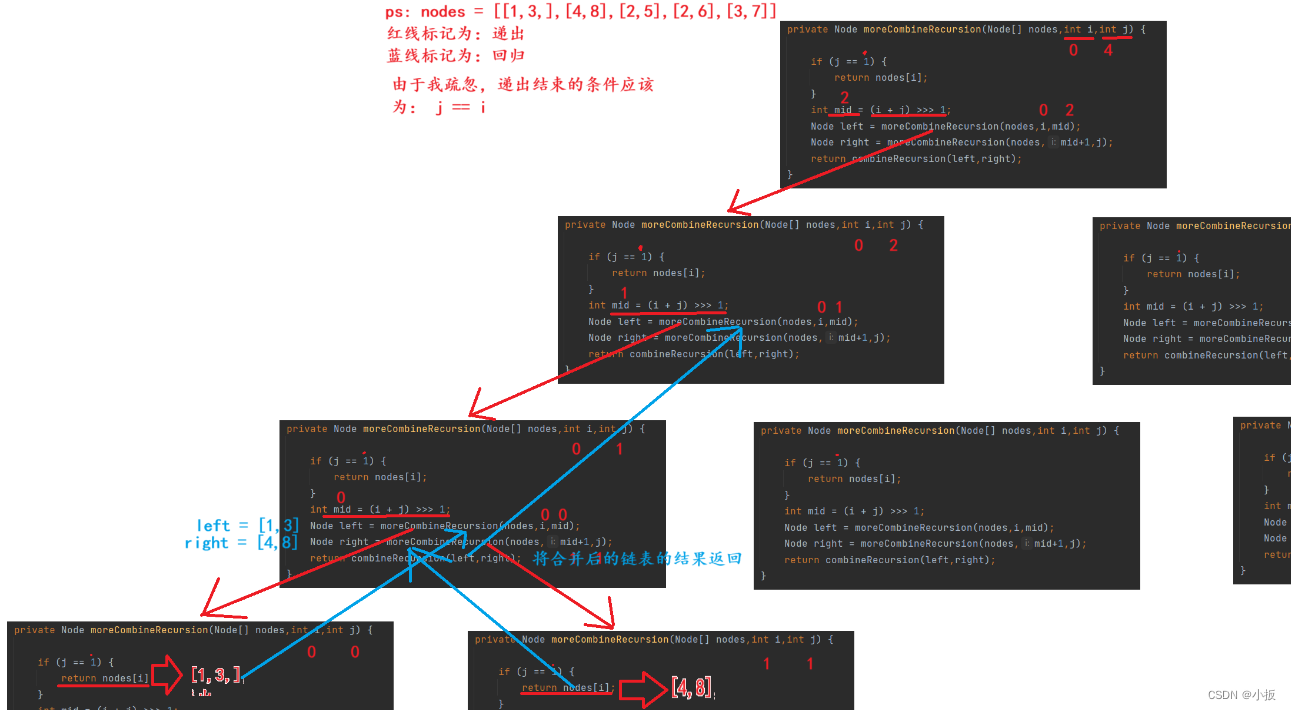
举例画图分析：

   ** 对以上的流程图简单分析：注意的是结束递出的条件为：i == j 结束递出，开始回归。回归的是每一个链表的节点，最后集齐了两个链表，需要通过利用两个链表升序合并的返回进行合并，可以用迭代法或者递归法。这只是其中的一小部分，不过每一个过程都是一样的，就不多赘述了。**

4.0 实现有序链表去重、合并升序链表的完整代码

import java.util.Iterator;

public class List implements Iterable<Integer>{
    private  Node sentry;

    static class Node {
        public int value;
        public Node next;

        public Node(int value, Node next) {
            this.value = value;
            this.next = next;
        }
    }

    public List() {
        sentry = new Node(-1,null);
    }

    //头插节点
    public void addFirst(int value) {
        sentry.next = new Node(value,sentry.next);
    }

    //尾插节点
    public void addLast( int value) {
        Node temp = sentry;
        while (temp.next != null) {
            temp = temp.next;
        }
        temp.next = new Node(value,null);
    }

    //重写迭代器
    @Override
    public Iterator<Integer> iterator() {
        return new Iterator<Integer>() {
            Node p = sentry.next;
            @Override
            public boolean hasNext() {
                return p != null;
            }

            @Override
            public Integer next() {
                int value = p.value;
                p = p.next;
                return value;
            }
        };
    }

    //去重方法二(保留):双指针
    public List removeDoublePointer(List list) {
        if (list == null) {
            return null;
        }

        //少于两个节点，不存在重复的值
        if (list.sentry.next == null || list.sentry.next.next == null) {
            return null;
        }

        Node n1 = list.sentry.next;
        Node n2;

        while ((n2 = n1.next) != null) {

            if (n2.value == n1.value) {
                n1.next = n2.next;
            }else {
                n1 = n1.next;
            }
        }

        return list;
    }


    //去重方法一(保留):递归
    public List removeRecursion(List list) {
        Node sentry1 = list.sentry;
        sentry = recursion1(sentry1);
        return list;
    }

    private Node recursion1(Node p) {

        if (p == null || p.next == null) {
            return p;
        }
        if (p.value == p.next.value) {
            return recursion1(p.next);
        }else {
            p.next = recursion1(p.next);
            return p;
        }

    }



    //去重方法一(不保留):递归
    public List removeRepeat(List list) {
        Node temp = list.sentry;
        sentry = recursion(temp);
        return list;
    }

    public Node recursion(Node p) {
        if (p == null || p.next == null) {
            return p;
        }

        if (p.value != p.next.value) {
            p.next = recursion(p.next);
            return p;
        }else {
            while (p.value == p.next.value) {
                p = p.next;
            }
            return recursion(p.next);
        }

    }


    //去重方法二(不保留):三指针
    public List removeThreePointer(List list) {

        if (list == null) {
            return null;
        }

        Node n1 = list.sentry;
        Node n2 ;
        Node n3 ;
        while ((n2 = n1.next) != null && (n3 = n2.next) != null) {
            if (n2.value == n3.value) {
                while (n3 != null && n2.value == n3.value) {
                    n3 = n3.next;
                }
                n1.next = n3;
            }else {
                n1 = n1.next;
            }
        }
        return list;
    }


    //合并升序链表
    public static List combinedList(List l1,List l2) {
        if (l1 == null && l2 == null) {
            return null;
        } else if (l1 == null) {
            return l2;
        } else if (l2 == null) {
            return l1;
        }

        List newList = new List();
        Node node1 = l1.sentry.next;
        Node node2 = l2.sentry.next;
        Node p = newList.sentry;

        while (node1 != null && node2 != null) {

            if (node1.value < node2.value) {
                p.next = node1;
                node1 = node1.next;
            }else {
                p.next = node2;
                node2 = node2.next;
            }
            p = p.next;
        }

        if (node1 != null) {
            p.next = node1;
        }
        if (node2 != null) {
            p.next = node2;
        }
        return newList;
    }

    //合并链表:递归实现
    public List combineList(List list2) {
        List newList = new List();
        Node p1 = this.sentry.next;
        Node p2 = list2.sentry.next;
        Node p = combineRecursion(p1,p2);
        newList.sentry.next = p;
        return newList;
    }

     private Node combineRecursion(Node p1, Node p2) {
        if (p1 == null) {
            return p2;
        } else if (p2 == null ) {
            return p1;
        }

        if (p1.value < p2.value) {
            p1.next = combineRecursion(p1.next,p2);
            return p1;
        }else {
            p2.next = combineRecursion(p1,p2.next);
            return p2;
        }

     }

     //实现多个升序链表合并
    public List moreCombine(Node[] nodes) {
        List list = new List();
        list.sentry.next = moreCombineRecursion(nodes,0, nodes.length-1);
        return list;
    }

    private Node moreCombineRecursion(Node[] nodes,int i,int j) {

        if (j == i) {
            return nodes[i];
        }
        int mid = (i + j) >>> 1;
        Node left = moreCombineRecursion(nodes,i,mid);
        Node right = moreCombineRecursion(nodes,mid+1,j);
        return combineRecursion(left,right);
    }

}